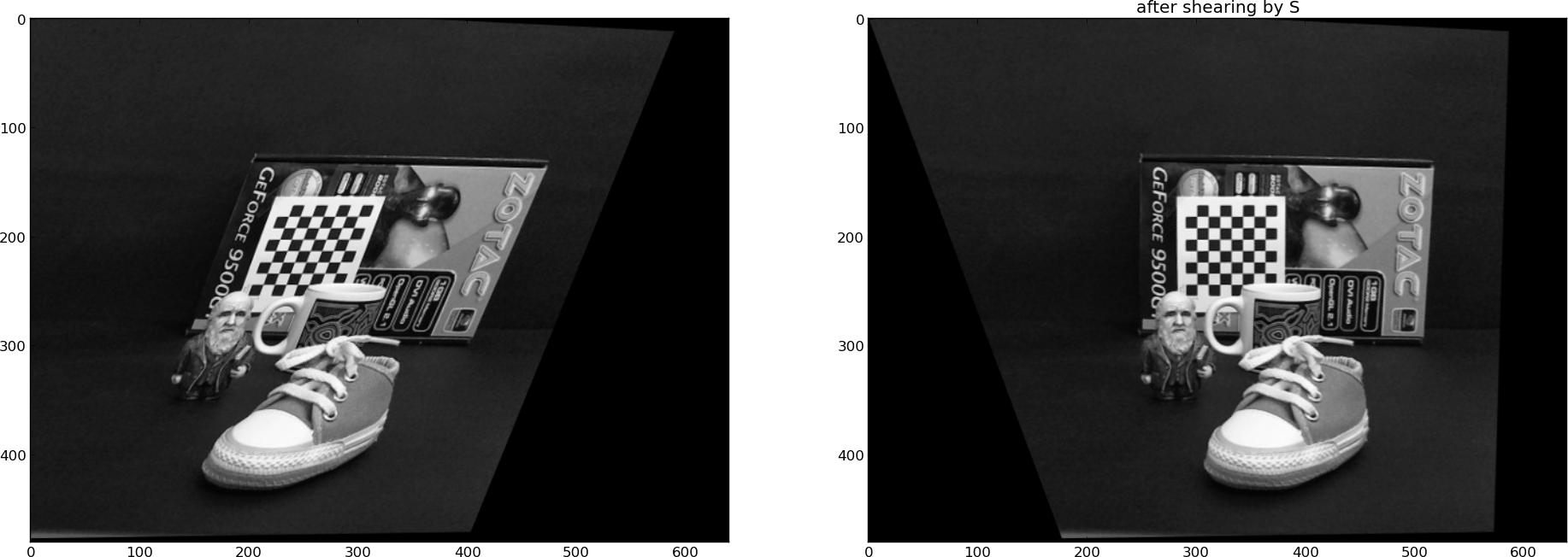
Heavy shearing effects using Hartley's rectification

asked 2012-07-16 15:08:20 -0600

Thiago Santos
141 ●4 ●9

I'm using stereoRectifyUncalibrated, OpenCV implementation for Richard Hartley's rectification algorithm. The problem is I'm observing shearing in one of the rectified images and it's causing problems on disparity computation.

Consider these two input images (the points pairs were produced using SURF):

Input images plus the SURF features

Hartley's algorithm produced these two rectified images:

Rectified images

The epipolar lines look fine. The following images shows some epipolar lines and a few point pairs:

Rectified epipolar lines

The rectified SURF keypoints look fine too, consider the following small sample for inspection, d is disparity and erro is the difference between the y coordinate (zero for a perfect mapping):

(306.28, 139.00) <-> (284.15, 138.48): d = -22.13, erro = -0.52
(259.84, 150.72) <-> (234.34, 150.51): d = -25.50, erro = -0.21
(423.93, 151.01) <-> (425.24, 150.71): d = 1.30, erro = -0.30
(220.98, 151.05) <-> (190.53, 151.05): d = -30.45, erro = -0.00
(354.21, 157.88) <-> (346.19, 157.91): d = -8.02, erro = 0.04
(304.17, 161.58) <-> (289.66, 161.80): d = -14.51, erro = 0.22
(229.47, 162.44) <-> (203.86, 162.27): d = -25.61, erro = -0.17
(406.54, 262.40) <-> (442.38, 262.91): d = 35.84, erro = 0.50
(361.67, 290.02) <-> (399.54, 289.98): d = 37.87, erro = -0.04
(356.44, 293.49) <-> (394.51, 292.96): d = 38.07, erro = -0.53
(340.01, 339.44) <-> (318.47, 339.75): d = -21.54, erro = 0.31
(245.47, 360.89) <-> (204.93, 360.18): d = -40.55, erro = -0.71
...

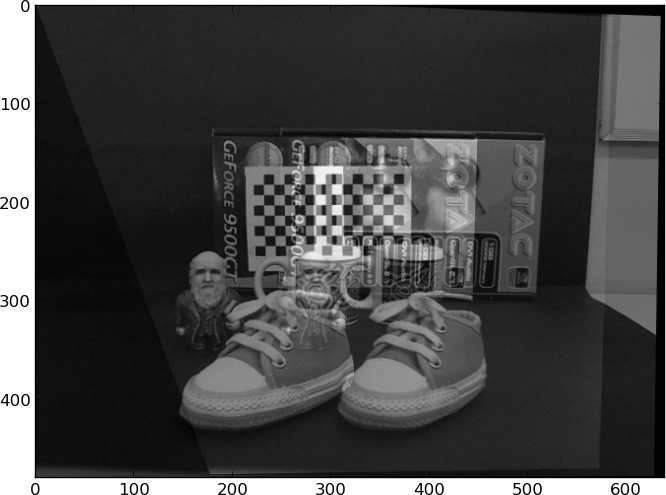
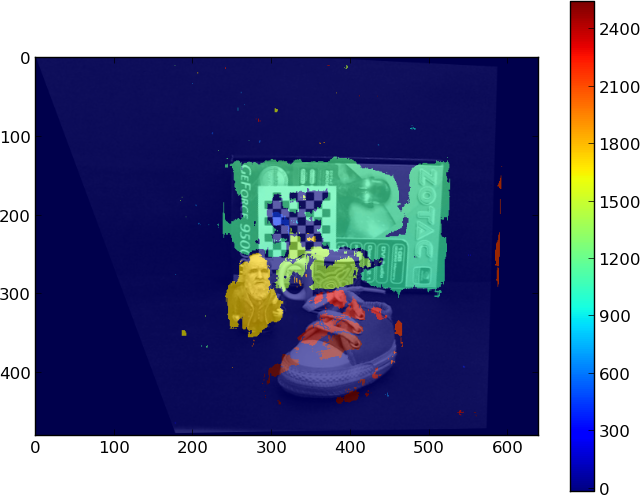
Now, the problem. The following image shows the two rectified images overlaped:

image description

The ZOTAC word printed in the box is a good example. The word is in the same plane and, ideally, should present similar disparity. But the observed shearing will produce small disparities for "Z pixels" compared to disparities for "C pixels".

Actually, it's not an OpenCV issue. I implemented Hartley's algorithm from scratch using Python and NumPy and got the same results. What is the matter with Hartley's algorithm? Can StereoBM handle this problem? Or have I made some mistake?

edit retag flag offensive close merge delete

add a comment

2 answers

Sort by » oldest newest most voted

answered 2012-07-23 11:26:38 -0600

Thiago Santos
141 ●4 ●9

Loop & Zhang present a solution in this paper. They use a shearing transform to reduce the distortion introduced by the projective transform that mapped the epipoles to infinity (ie, that made the epipolar lines parallel). Consider the shearing transform

     | k1 k2 0 |
S =  | 0   1 0 |.
     | 0   0 1 |

Let w and h be image width and height respectively. Consider the four midpoints of the image edges:

a = ((w-1)/2, 0, 1),
b = (w-1, (h-1)/2, 1),
c = ((w-1)/2, h-1, 1) and
d = (0, (h-1)/2, 1).

According to Loop & Zhang:

(...) we attempt to preserve perpendicularity and aspect ratio of the lines bd and ca

Let H be the rectification homography and let a' = Ha be a point in the affine plane by dividing through so that a'[2] = 1 (note a'[2] is the third component, ie, a' = (a'[0], a'[1], a'[2])). Let

x = b' - d',
y = c' - a'

According to Loop & Zhang:

As the difference of affine points, x and y are vectors in the euclidean image plane. Perpendicularity is preserved when (Sx)^T(Sy) = 0, and aspect ratio is preserved if [(Sx)^T(Sx)]/[(Sy)^T(Sy)] = w²/h².

The real solution presents a closed-form:

k1 = (h²x[1]² + w²y[1]²)/(hw(x[1]y[0] - x[0]y[1])) and
k2 = (h²x[0]x[1] + w²y[0]y[1])/(hw(x[0]y[1] - x[1]y[0]))

up to sign (the positive is preferred).

This is the result after applying shearing S on the left image:

image description

Now the left and right images overlaped:

image description

Finally, OpenCV stereo method StereoBM can compute a reasonable result for disparity:

image description

edit flag offensive delete link

Comments

Hi, I recently met with the same problem. After stereoRectifyUncalibrated, the rectified images present severe shearing. I tried to implement the anti-shearing method you mentioned, but it seems the result is wrong. Could you share your images with me? I want to test whether it's the problem of images or program. Thanks!

meinvlv ( 2015-04-20 22:18:22 -0600 )edit

add a comment

answered 2012-07-18 02:07:56 -0600

Henrik Brauer
11 ●2

I am not exactly sure if I understood you correctly, but is your problem not exactly what Hartley discripes at page 12 with the block houses.

In this case, because of the wide difference in viewpoint, and the three-dimensional shape of the objects, the two images even after resampling look quite different.

edit flag offensive delete link

add a comment

Heavy shearing effects using Hartley's rectification

2 answers

Comments

Links

Question Tools

Stats

Related questions

Heavy shearing effects using Hartley's rectification edit

2 answers

Comments

Links

Question Tools

Stats

Related questions

Heavy shearing effects using Hartley's rectification